Binary Tree Zigzag Level Order Traversal
Problem Description
Given a binary tree, return the zigzag level order traversal of its nodes' values. (i.e., from left to right, then right to left for the next level and alternate between).
Examples
Example 1:
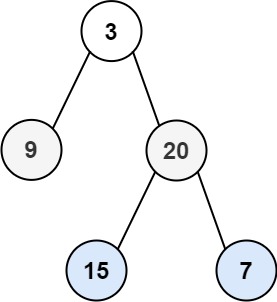
Input: root = [3,9,20,null,null,15,7]
Output: [[3],[20,9],[15,7]]
Constraints
- The number of nodes in the tree is in the range .
Solution for Binary Tree Problem
Intuition And Approach
To perform a zigzag level order traversal, we can use a breadth-first search (BFS) with a queue. We'll use a boolean flag to determine the direction of traversal at each level.
- Breadth-First Search (BFS): Traverse the tree level by level.
- Direction Toggle: Use a flag to toggle the direction of traversal for each level (left-to-right or right-to-left).
- Iterative Simulation
Code in Different Languages
- Java
- Python
- C++
class Solution {
public int minDepth(TreeNode root) {
if (root == null) return 0;
Queue<TreeNode> queue = new LinkedList<>();
queue.offer(root);
int depth = 1;
while (!queue.isEmpty()) {
int levelSize = queue.size();
for (int i = 0; i < levelSize; i++) {
TreeNode node = queue.poll();
if (node.left == null && node.right == null) return depth;
if (node.left != null) queue.offer(node.left);
if (node.right != null) queue.offer(node.right);
}
depth++;
}
return depth;
}
}
class Solution:
def minDepth(self, root):
if not root:
return 0
queue = collections.deque([(root, 1)])
while queue:
node, depth = queue.popleft()
if not node.left and not node.right:
return depth
if node.left:
queue.append((node.left, depth + 1))
if node.right:
queue.append((node.right, depth + 1))
class Solution {
public:
int minDepth(TreeNode* root) {
if (!root) return 0;
queue<TreeNode*> q;
q.push(root);
int depth = 1;
while (!q.empty()) {
int levelSize = q.size();
for (int i = 0; i < levelSize; ++i) {
TreeNode* node = q.front();
q.pop();
if (!node->left && !node->right) return depth; // Leaf node found
if (node->left) q.push(node->left);
if (node->right) q.push(node->right);
}
++depth;
}
return depth;
}
};
Complexity Analysis
- Time Complexity: where n is the number of nodes in the binary tree.
- Space Complexity: where h is the height of the binary tree.